Visual Aid Volunteers of Florida, Inc.

As we all know, the dot 5 in Nemeth transcriptions represents both the baseline indicator and the broadly named multipurpose indicator. The central usage of either of these symbols is to denote a return to the baseline from a superscripted or subscripted item or to indicate the beginning of a modified expression. However, as its name implies, the multipurpose indicator serves additional functions beyond this limited scope. We are going to take a brief tour of some of the multipurpose indicator's other, perhaps rarer, usages and functions.

A central secondary function of the multipurpose indicator is to indicate non-simultaneous, or horizontal, print elements. The most common instance of this function, which occurs with some degree of regularity, would be a non-simultaneous plus and minus. So as not to be confused with the plus-over-minus or minus-over-plus print symbol, the multipurpose indicator is needed between these two symbols when they follow each other in print.

For example, while

2x ± 3x + y

would be transcribed as

_% #2x+-3x+y _:,

if this same example was depicted in print as

2x + -3x + y,

the multipurpose indicator would need to be used to properly illustrate the operators' non-simultaneous print layout (and differing meaning), and therefore would be rendered in braille as

_% #2x+"-3x+y _:.

As with non-simultaneous operators, comparison signs compounded horizontally use the multipurpose indicator to distinguish them from vertically aligned comparison signs. As these compounded symbols comprise a single comparative unit, no spacing should be added within the symbols themselves. Thus, the passage

a <=> b

would be represented in braille as:

_% a "k".k".1 b _:.

When arrows are used in a mathematical context as comparison signs, they would likewise require the multipurpose indicator. (However, if the subject is chemistry, alternate symbols apply.) An example of compounded arrows (within a shape) will be dealt with shortly.

As with the above examples, distinction must be made between a horizontal double tilde and a vertical double tilde due to their respective meanings (the former an operator meaning "not," and the latter a sign of comparison meaning "approximately equal to"). For example, if the following—and admittedly highly unlikely—print expression were to occur, the different meanings of both signs would be needed to be properly reflected in braille.

~~p ≈ q ∧ r

As such, the braille would be interpreted in braille as:

_% @:"@:P @:@: q@%r _:.

Another instance of non-simultaneous usage occurs with either non-simultaneous subscripts and superscripts or between an unspaced right subscript/superscript and an unspaced left subscript/superscript pertaining to two separate elements. Regarding non-simultaneous subscripts or superscripts, while

x²₁

_% x1^2 _:,

x²₁

_% x^2";1 _:.

Likewise, when the scripted elements pertain to two different items, the multipurpose indicator must be used to distinguish their component parts. Consequently,

p^b _cq

would be produced in braille as:

_% p^b";c"q _:.

In the above, note that even if print depicts a space between these elements for clarity for the print reader, that space is omitted in braille. The multipurpose indicator makes clear the distinction and better reflects the expression's intent.

For the final example of non-simultaneous usage and the multipurpose indicator, we turn to shapes with interior modification. As the arrows are not vertically aligned in the following shape, we must note that in the braille with the usage of the multipurpose indicator. As a result, the print illustration shown here:

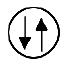
would be represented in braille as:

_% $c_$$%33o"$<33o] _:.

The multipurpose indicator is typically used in the interests of clarity or exacting presentation of print symbols, as was shown with the non-simultaneous examples above, because meaning changes with presentation of mathematical symbols. Another purpose that serves the interests of clarity is to distinguish symbols from one another, i.e., where one symbol ends and another begins, as is the common case with adjacent vertical bars and adjacent double vertical bars. So, if we have two adjacent vertical bars like this:

|x||y|,

the braille would need a multipurpose indicator to let the reader know the middle bars are two distinct symbols and not a double vertical bar, and would be rendered in braille as:

_% \x\"\y\ _:.

As the guidebooks note, care must be taken to ensure the symbols are indeed separate entities and not a double vertical bar. Print will often use identifiers to signify this distinction utilizing differing bar types, such as thicker bars and/or longer or shorter bars. A double vertical bar is very often bolded in print as well.

Now that we have the non-simultaneous and horizontally positioned items out of the way, we can turn to a few of the multipurpose indicator's other functions. As the guidance documents attest, another of its primary purposes is to indicate when a numeral adjacent to a letter (from any alphabet) is not in the subscript position. Thus, if we have a division remainder of 3, R3 would be brailled as:

_% ,r"3 _:

This equally applies when the number is a decimal. R.24 would be brailled as:

_% ,R".24 _:

On the topic of decimal points, the multipurpose indicator plays an outsized role with them when non-numeric elements follow a decimal point. This includes anything non-numeric (excepting the punctuation indicator and the mathematical comma), be they letters, operators, signs of omission, mathematical parentheses, etc. I will limit myself to only a few examples of these for brevity's (lamented) sake.

For example, a multipurpose indicator would be needed when a decimal point is followed by a fraction line or closing fraction indicator, as indicators are non-numeric. Thus,

$\frac{\mathrm{2.}}{\mathrm{3.}}$

would be brailled as:

_% ?2."/3."# _:.

Perhaps the most common instance of encountering the decimal issue would concern blank lines for answers. For example, if we have

3.9-2.2= 1.____,

it would be brailled as:

_% #3.9-2.2 .k #1."---- _:

(Reminder that the required spacing around the long dash does not apply to decimal points.)

Just to note, however, if the blank answer does not contain a numeral, the expression should not have a number sign. So, if our equation above were

3.9-3.2= .____,

it would be brailled as:

_% #3.9-3.2 .k ."---- _:.

Moving on from decimals, another sign of omission that requires a multipurpose indicator would be any of the regular polygons that are expressed by the shape indicator and a number denoting the number of sides they contain. (One can only assume this is to avoid a misreading of the following 4-sided polygon as a 44-sided polygon, which would be a monumental polygon indeed!) Thus,

9◻4 = 13

would be expressed in braille thusly:

_% #9$4"4 .k #13 _:.

While at the outset, I promised this brief article would focus on the dot 5 beyond its usage in modified expressions and as the baseline indicator, it should be known I am at times an unreliable narrator. Thus, we will now examine a case in which the multipurpose indicator for modified expressions, the multipurpose indicator for clarity, and the baseline indicator are all required.

In the following example

₁A_x̃^yB_{x̃ + ỹ}

baseline indicators are needed following the termination of each subscript and superscript, the multipurpose indicator is needed between the first subscripted x-tilde and the superscripted y to ensure the reader understands these are not simultaneous scripts, and the multipurpose indicator must also be used for each of the three modified expressions. (Also note the usage of a repeated subscript indicator prior to the second multipurpose indicator for the y-tilde modified expression so that the multipurpose indicator is not mistaken for a baseline indicator.) Taken in whole, the braille for this would be presented as follows:

_% ;1",a;"x<@:]"^y",b;"x<@:]+;"y<@:] _:.

While this article touched upon some of the further attributes of the multipurpose indicator, it is far from a comprehensive examination. I urge the reader, if interested, to avail themselves of the recently released/updated guidance documents, The Nemeth Braille Code for Mathematics and Science Notation, 2022, and An Introduction to Braille Mathematics Using UEB with Nemeth, if they wish to learn more. These can be found on the BANA and NFB websites.